Video on Profit and Loss - shortcuts, tips and tricksProfit and Loss
Important Terms: Cost Price (C.P.): Price at which at an article is purchased.
Selling Price (S.P.): Price at which at an article is sold
Profit/Gain: The seller is said to be in profit, if selling price (S.P.) is greater than cost price (C.P.)
Loss: The seller is said to be in loss, if selling price (S.P.) is lesser than cost price (C.P.)
Important Formulae: | 1) Loss% = | Loss | × 100 |
| C.P. |
| 2) Profit% = | Profit | × 100 |
| C.P. |
3) When shopkeeper earns profit, | Cost Price = | 100 | × S.P. - - - - - - (Given: Gain% and selling price of an article) |
| (100 + Gain%) |
| Selling Price = | (100 + Gain%) | × C.P. - - - - - - (Given: Gain% and cost price of an article) |
| 100 |
4) When shopkeeper incurs loss, | Cost Price = | 100 | × S.P. - - - - - - (Given: Loss% and selling price of an article) |
| (100 - Loss%) |
| Selling Price = | (100 - Loss%) | × C.P. - - - - - - (Given: Loss% and cost price of an article) |
| 100 |
Quick Tips and Tricks1) Profit = (S.P.) > (C.P.)
2) Loss = (S.P.) < (C.P.)
3) If profit earned by selling an article is 25 %, then S.P. = 125% of C.P.
4) If an article is sold at a loss of 30 %, then S.P. = 70 % of C.P.
5) A shopkeeper sells two similar items A and B. If A is sold at a gain of x % and B is sold at a loss of x %, then shopkeeper always incurs a loss given by:
| Loss% = |  | Common loss and gain% |  | 2 | = |  | x |  | 2 |
| 10 | | 10 |
6) A trader sells goods at cost price but uses a weight of
x kg instead of y kg (false weights) and makes profit. This profit can be calculated using the formula shown below:
True weight – False weight = Error | Gain% = |  | Error | × 100 |  | % |
| (True weight – Error) |
7) If cost price of X articles is equal to selling price of Y articles, then profit can be calculated using the formula:
a) C.P. of X = S.P. of Yb) Number of X articles > Number of Y articles| Profit% = | No. of X articles – No. of Y articles | × 100 |
| No. of Y articles |
8) If a seller makes X % above C.P. and offers a discount of Y%, then profit % or loss % can be calculated using the formula:
| Profit or Loss% = (X – Y) – | X × Y |
| 100 |
9) Discount: | a) Discount% = | Discount | × 100 |
| Marked Price (M.P.) |
| b) If D1, D2, D3 are percentage of successive discounts on M.P., then S.P. | = | Marked Price | 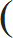 | 1 – | D1 | 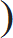 | 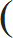 | 1 – | D2 | 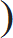 | 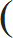 | 1 – | D3 | 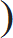 |
| 100 | 100 | 100 |
c) If a and b are two successive discount percentages, then single equivalent discount percentage is given as:
| Single equivalent discount percentage = |  | (a + b) – | ab |  |
| 100 |
Question Variety Type 1: To find Profit/Loss or Selling Price/Cost Price.
A shopkeeper sells an article for Rs. 200 with a loss of Rs. 20 %. Find the cost price of the article.
a. 220
b. 250
c. 280
d. 260
View solution Correct Option: (b)
Hint:
| Cost Price = | 100 | × S.P. |
| (100 – Loss%) |
We are given that,
S.P. = Rs. 200 and loss = 20
| Cost Price = | 100 | × 200 |
| (100 – 20) |
Cost Price = Rs. 250A trader expects a gain of 15 % on his cost price. If in a week his sale is of Rs. 580, then what is his profit?
a. 75.65
b. 73.26
c. 72.50
d. 70.78
View solution Correct Option: (a)
We are given selling price = Rs. 580 and expected profit of 15 %
Therefore, we can easily solve this numerical, considering basic formulae of profit and loss.
Let cost price = x
Selling price = C.P. + Profit
S.P. = C.P. + (15% of C.P.) ---------- (We know that profit is gained on cost price)
580 = x + (0.15 x)
580 = 1.15 x
Therefore,
x = 504.347
Cost Price = Rs. 504.35
Now, we have the cost price and hence,
Profit = S. P. – C.P. = 580 – 504.35 = Rs. 75.65
The trader gets a profit of Rs. 75.65
Alternate solution: This numerical can be easily solved using the trick specified below. Using such tricks reduce time consumed to solve the numerical.
Hint:
| Cost Price = | 100 | × S.P. |
| (100 + Gain%) |
| Cost Price = | 100 | × 580 |
| (100 + 15) |
| Cost Price = | 100 | × 580 = Rs. 504.35 |
| (115) |
Therefore,
Profit = C.P. – S.P.
C.P. = Rs.504. 35 and S.P. = Rs. 580
Profit = 580 – 504.35 = Rs. 75.65
The trader gets a profit of Rs. 75.65If a boy sells a book for Rs. 450 he gets a loss of 10 %, then find cost price. To gain 10 %, what should be the selling price?
a. 400, 500
b. 550, 600
c. 500, 550
d. 475, 525
View solution Correct Option: (c)
1) Find cost price
Let C.P. of book = x and S.P. = Rs. 450
S.P. of book = C.P. – (10% of C.P.)
S.P. = x – (0.10x)
450 = 0.9 x
x i.e cost price = Rs. 500
2) Find Selling Price to gain 10 %.
Now, we are asked to find selling price to gain 10% profit.
Hint:
| Selling Price = | (100 + Gain%) | × C.P. |
| 100 |
| Selling Price = | (100 + 10) | × 500 |
| 100 |
| Selling Price = | (110) | × 500 |
| 100 |
Therefore, selling Price = Rs. 550A merchant sells 30 metres of cloth and gains selling price of 10 metres. Find the gain percent.
a. 15 %
b. 25 %
c. 50 %
d. 75%
View solution Correct Option: (c)
Here, selling price of 10 m cloth is obtained as profit.
Profit of 10 m cloth = (S.P. of 30 m cloth) – (C.P. of 30 m cloth)
Selling price of 20 m cloth = Selling Price of 30 m of cloth
Let cost of each metre be Rs. 100.
Therefore, cost price of 20 m cloth = Rs. 2000 and S.P. of 20 m cloth = Rs. Rs. 3000
| Profit% = | 10 | × 100 = 50% |
| 20 |
Profit of 50 % was made by the merchant.